Introduction:
Students who are ready for Geometry have successfully completed Pre-Algebra (generally an eighth-grade math course). It is highly recommended that Algebra 1 be completed as well.
Preparing for the Assessment:
You will need the following items:
- pencil with eraser
- extra paper for calculation, if desired
Students may also use a calculator to complete this assessment.
Gather these materials before you begin.
Student Portion:
Print out a copy of the Geometry Readiness Assessment. The printed part of the assessment is to be completed independently by the student and should take approximately 30 minutes. Be sure to keep track of the actual time your student spends on this part of the assessment. You may attempt to clarify the wording of a question if your student does not understand, but you should not answer specific questions asking how to solve a particular problem.
When your student has completed his work on paper, come back to the computer to complete the rest of the assessment.
Instructor Portion:
Use this tool as an opportunity to help you determine your student’s understanding of the concepts.
For each problem, first check to see if the student answered it correctly. Then ask your student to explain to you how he arrived at each of his answers, or “teach back” the solution. Based on your student’s response, choose the statement(s) that most accurately describe how your student solved the problem. (IMPORTANT: Several of the questions require multiple responses. Be sure to mark ALL appropriate responses.)
Quiz-summary
0 of 12 questions completed
Questions:
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
Information
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading…
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
Results
Results
Time has elapsed
Categories
- Not categorized 0%
-
Your student’s performance indicates that he would be more successful in a lower level. Here are some additional steps you can take.
-
Your student’s performance indicates that he may be ready for Geometry. Here are some additional steps you should take.
-
Your student is ready for Geometry. Please be advised, however, that many students complete the Math-U-See Algebra 1 course before moving into Geometry. You will see review problems from Algebra 1 on Geometry tests, but you may omit them if your student has not yet covered the material.
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- Answered
- Review
- Question 1 of 12
1. Question
CORRECT ANSWER:
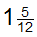
SAMPLE SOLUTIONS:
Solution A
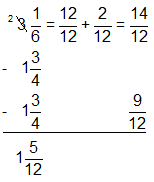
Solution B
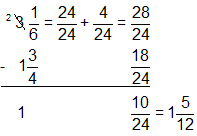
Ask the student to explain how he found the answer. Which of the following statements characterized his response? CLICK ALL THAT APPLY.
CorrectCORRECT ANSWER:
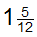
SAMPLE SOLUTIONS:
Solution A
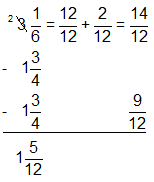
Solution B
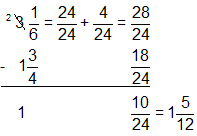
The student needed to know how to subtract mixed numbers that require regrouping (borrowing). This includes all of the following:
1) Finding a common denominator
2) Regrouping (borrowing) from the whole number
3) Subtracting the fractions and subtracting the whole numbers
4) Simplifying the answer, if necessary (not needed in this particular problem)SKILL: Subtract mixed numbers with unlike denominators and simplify the answer to lowest terms.
IncorrectCORRECT ANSWER:
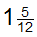
SAMPLE SOLUTIONS:
Solution A
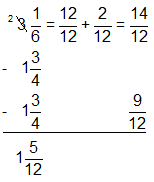
Solution B
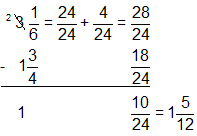
The student needed to know how to subtract mixed numbers that require regrouping (borrowing). This includes all of the following:
1) Finding a common denominator
2) Regrouping (borrowing) from the whole number
3) Subtracting the fractions and subtracting the whole numbers
4) Simplifying the answer, if necessary (not needed in this particular problem)SKILL: Subtract mixed numbers with unlike denominators and simplify the answer to lowest terms.
- Question 2 of 12
2. Question
CORRECT ANSWER: 2
SAMPLE SOLUTIONS:
Solution A

Solution B

Solution C
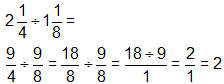
Ask the student to explain how he found the answer. Which of the following statements characterized his response? CLICK ALL THAT APPLY.
CorrectCORRECT ANSWER: 2
This problem could be solved in one of three ways.
Solution A
1) Change the mixed numbers to improper fractions.
2) Multiply by the reciprocal of the second factor.
3) Divide the common factors.
4) Multiply the numerators and denominators.

Solution B
1) Change the mixed numbers to improper fractions.
2) Multiply by the reciprocal of the second factor.
3) Divide the answer by the Greatest Common Factor (GCF) or a common factor.

Solution C
1) Change the mixed numbers to improper fractions.
2) Find a common denominator.
3) Divide the numerators and then divide the denominators.
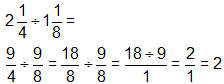
SKILL: Divide mixed numbers and simplify the answer to lowest terms.
IncorrectCORRECT ANSWER: 2
This problem could be solved in one of three ways.
Solution A
1) Change the mixed numbers to improper fractions.
2) Multiply by the reciprocal of the second factor.
3) Divide the common factors.
4) Multiply the numerators and denominators.

Solution B
1) Change the mixed numbers to improper fractions.
2) Multiply by the reciprocal of the second factor.
3) Divide the answer by the Greatest Common Factor (GCF) or a common factor.

Solution C
1) Change the mixed numbers to improper fractions.
2) Find a common denominator.
3) Divide the numerators and then divide the denominators.
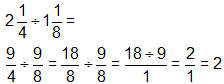
SKILL: Divide mixed numbers and simplify the answer to lowest terms.
- Question 3 of 12
3. Question
CORRECT ANSWER: 134
Ask the student to explain how he found the answer. Which of the following statements characterized his response? CLICK ALL THAT APPLY.
CorrectCORRECT ANSWER: 134
The student needed to know that exponents represent repeated multiplication. The base (13) is being multiplied by itself four times, so the student would write a raised number 4 to show this.
SKILL: Use an exponent to rewrite an expression with repeated multiplication.
IncorrectCORRECT ANSWER: 134
The student needed to know that exponents represent repeated multiplication. The base (13) is being multiplied by itself four times, so the student would write a raised number 4 to show this.
SKILL: Use an exponent to rewrite an expression with repeated multiplication.
- Question 4 of 12
4. Question
CORRECT ANSWER:
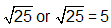
Ask the student to explain how he found the answer. Which of the following statements characterized his response? CLICK ALL THAT APPLY.
CorrectCORRECT ANSWER:
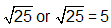
The student was expected to know a radical is a root. In this case, 5 is the square root of 25, written as shown.
SKILL: Use a radical to show the relationship between a square and its root.
IncorrectCORRECT ANSWER:
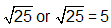
The student was expected to know a radical is a root. In this case, 5 is the square root of 25, written as shown.
SKILL: Use a radical to show the relationship between a square and its root.
- Question 5 of 12
5. Question
CORRECT ANSWER: x = 1.8
SAMPLE SOLUTIONS:
Solution A:

Solution B:
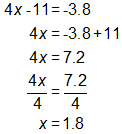
Solution C:

Ask the student to explain how he found the answer. Which of the following statements characterized his response? CLICK ALL THAT APPLY.
CorrectCORRECT ANSWER: x = 1.8
The student needed to solve for the unknown (x). He could have done this in one of three ways:
Solution A:
1) Multiply all terms by 10 so that there are no decimals.
2) Add 110 to both sides to isolate the term with the variable.
3) Divide both sides by the coefficient, 40 (or multiply by the reciprocal, 1/40).

Solution B:
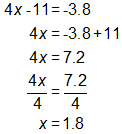
1) Add 11 to both sides to isolate the term with the variable.
2) Divide both sides by the coefficient, 4.
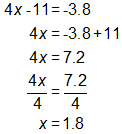
Solution C:
1) Add 11 to both sides to isolate the term with the variable.
2) Multiply both sides of the equation by the reciprocal of the coefficient, 1/4.

SKILL: Solve a two-step equation with one unknown.
IncorrectCORRECT ANSWER: x = 1.8
The student needed to solve for the unknown (x). He could have done this in one of three ways:
Solution A:
1) Multiply all terms by 10 so that there are no decimals.
2) Add 110 to both sides to isolate the term with the variable.
3) Divide both sides by the coefficient, 40 (or multiply by the reciprocal, 1/40).

Solution B:
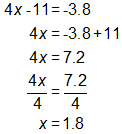
1) Add 11 to both sides to isolate the term with the variable.
2) Divide both sides by the coefficient, 4.
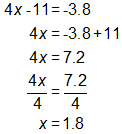
Solution C:
1) Add 11 to both sides to isolate the term with the variable.
2) Multiply both sides of the equation by the reciprocal of the coefficient, 1/4.

SKILL: Solve a two-step equation with one unknown.
- Question 6 of 12
6. Question
CORRECT ANSWER: x = 38
SAMPLE SOLUTIONS:
Solution A:

Solution B:
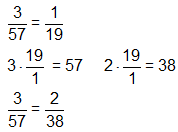
Solution C:
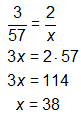
Ask the student to explain how he found the answer. Which of the following statements characterized his response? CLICK ALL THAT APPLY.
CorrectCORRECT ANSWER: x = 38
The student needed to know how to solve for an unknown in a proportion. This could have been done in one of three ways:
Solution A:
1) Determine the ratio between the two known numerators (in this case, 2/3).
2) Multiply the numerator and denominator of the known fraction (3/57) by that ratio.

Solution B:
1) Determine the ratio between the numerator and denominator of the given fraction (in this case, 1/19).
2) Multiply both numerators by the reciprocal of that ratio (19/1).
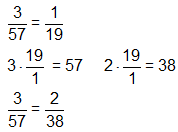
Solution C:
1) Cross multiply the numerators and denominators.
2) Solve for the unknown.
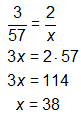
SKILL: Solve for the unknown in a proportion.
IncorrectCORRECT ANSWER: x = 38
The student needed to know how to solve for an unknown in a proportion. This could have been done in one of three ways:
Solution A:
1) Determine the ratio between the two known numerators (in this case, 2/3).
2) Multiply the numerator and denominator of the known fraction (3/57) by that ratio.

Solution B:
1) Determine the ratio between the numerator and denominator of the given fraction (in this case, 1/19).
2) Multiply both numerators by the reciprocal of that ratio (19/1).
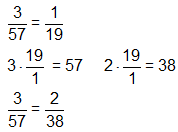
Solution C:
1) Cross multiply the numerators and denominators.
2) Solve for the unknown.
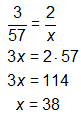
SKILL: Solve for the unknown in a proportion.
- Question 7 of 12
7. Question
CORRECT ANSWER: 350 calories
SAMPLE SOLUTIONS:
Solution A:
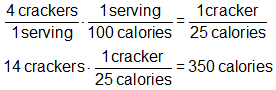
Solution B:
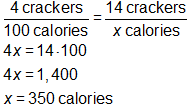
Solution C:
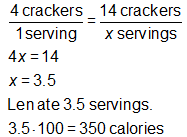
Ask the student to explain how he found the answer. Which of the following statements characterized his response? CLICK ALL THAT APPLY.
CorrectCORRECT ANSWER: 350 calories
Math-U-See emphasizes the application of mathematical knowledge to solve real-world problems. This particular problem required applying an understanding of ratios and may have resulted in one of the following sample solutions:
Solution A:
1) Write a ratio for the number of crackers in a serving.
2) Multiply by a unit multiplier (a ratio showing the number of calories in a serving) to find the number of calories in one cracker.
3) Multiply the number of crackers by the number of calories in a cracker to arrive at the total.
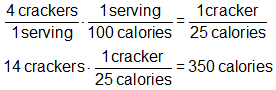
Solution B:
1) Write ratios for the number of calories in a serving (4 crackers) and a ratio for the missing information.
2) Set up a proportion with the two ratios and solve. (This sample solution shows solving by cross multiplying.)
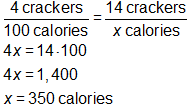
Solution C:
1) Write ratios for the number of calories in a serving (4 crackers) and a ratio for the missing information.
2) Set up a proportion with the two ratios and solve. (This sample solution shows solving by finding the ratio of the known numerators.)
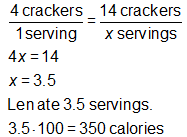
SKILL: Solve a word problem involving ratios.
IncorrectCORRECT ANSWER: 350 calories
Math-U-See emphasizes the application of mathematical knowledge to solve real-world problems. This particular problem required applying an understanding of ratios and may have resulted in one of the following sample solutions:
Solution A:
1) Write a ratio for the number of crackers in a serving.
2) Multiply by a unit multiplier (a ratio showing the number of calories in a serving) to find the number of calories in one cracker.
3) Multiply the number of crackers by the number of calories in a cracker to arrive at the total.
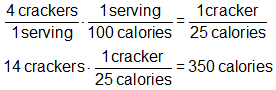
Solution B:
1) Write ratios for the number of calories in a serving (4 crackers) and a ratio for the missing information.
2) Set up a proportion with the two ratios and solve. (This sample solution shows solving by cross multiplying.)
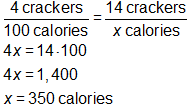
Solution C:
1) Write ratios for the number of calories in a serving (4 crackers) and a ratio for the missing information.
2) Set up a proportion with the two ratios and solve. (This sample solution shows solving by finding the ratio of the known numerators.)
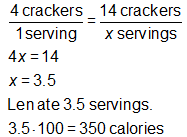
SKILL: Solve a word problem involving ratios.
- Question 8 of 12
8. Question
CORRECT ANSWER: A matches Y, B matches X, and C matches Z.
Ask the student to explain how he found the answer. Which of the following statements characterized his response? CLICK ALL THAT APPLY.
CorrectCORRECT ANSWER: A matches Y, B matches X, and C matches Z.
This problem assessed the student
IncorrectCORRECT ANSWER: A matches Y, B matches X, and C matches Z.
This problem assessed the student
- Question 9 of 12
9. Question
CORRECT ANSWER: Quadrant II
Ask the student to explain how he found the answer. Which of the following statements characterized his response? CLICK ALL THAT APPLY.
CorrectCORRECT ANSWER: Quadrant II
The student needed to know the four quadrants of the coordinate grid, which are identified with Roman numerals.

In addition, he needed to be able to identify the location of the point after it was moved. The new point is represented as C’ in the graphic above. Since the first coordinate is negative and the second is positive, the point would be located in Quadrant II.
SKILL: Identify the quadrants on a coordinate grid.
IncorrectCORRECT ANSWER: Quadrant II
The student needed to know the four quadrants of the coordinate grid, which are identified with Roman numerals.

In addition, he needed to be able to identify the location of the point after it was moved. The new point is represented as C’ in the graphic above. Since the first coordinate is negative and the second is positive, the point would be located in Quadrant II.
SKILL: Identify the quadrants on a coordinate grid.
- Question 10 of 12
10. Question
CORRECT ANSWER: He needs to use the 12-cm piece, the 9-cm piece, and the 6-cm piece.
Ask the student to explain how he found the answer. Which of the following statements characterized his response? CLICK ALL THAT APPLY.
CorrectCORRECT ANSWER: He needs to use the 12-cm piece, the 9-cm piece, and the 6-cm piece.
This particular problem assesses your student’s ability to solve a geometric problem logically, without drawing or resorting to trial and error. Students using logic to solve this problem would have arrived at these conclusions:
1) Using all four pieces: 12 cm + 10 cm + 9 cm + 6 cm = 37 cm (too long)
Using the longest two pieces: 12 cm + 10 cm = 22 cm (too short)
A combination of three pieces must be used.2) There were four different possible combinations using three pieces:
12 cm, 10 cm, 9 cm
12 cm, 10 cm, 6 cm
12 cm, 9 cm, 6 cm
10 cm, 9 cm, 6 cm3) Of the four combinations, only one gave a sum of 27 cm.
SKILL: Use logic to solve a real-world problem.
IncorrectCORRECT ANSWER: He needs to use the 12-cm piece, the 9-cm piece, and the 6-cm piece.
This particular problem assesses your student’s ability to solve a geometric problem logically, without drawing or resorting to trial and error. Students using logic to solve this problem would have arrived at these conclusions:
1) Using all four pieces: 12 cm + 10 cm + 9 cm + 6 cm = 37 cm (too long)
Using the longest two pieces: 12 cm + 10 cm = 22 cm (too short)
A combination of three pieces must be used.2) There were four different possible combinations using three pieces:
12 cm, 10 cm, 9 cm
12 cm, 10 cm, 6 cm
12 cm, 9 cm, 6 cm
10 cm, 9 cm, 6 cm3) Of the four combinations, only one gave a sum of 27 cm.
SKILL: Use logic to solve a real-world problem.
- Question 11 of 12
11. Question
The next two questions are just for the instructor. Click on the statement that best describes your student’s work during the written part of the assessment.
How long did it take the student to complete the assessment? Click on the best response.
CorrectA student who has mastered the prerequisite concepts should be able to complete the written assessment in about 45 minutes.
IncorrectA student who has mastered the prerequisite concepts should be able to complete the written assessment in about 45 minutes.
- Question 12 of 12
12. Question
How often did the student ask for help or hints as to how to solve a problem? (Note: this is different than asking for clarification about how a question is worded.) Click on the best response.
CorrectA student who has mastered the prerequisite concepts should feel confident in his or her ability to solve the problems and should not need to ask for assistance.
IncorrectA student who has mastered the prerequisite concepts should feel confident in his or her ability to solve the problems and should not need to ask for assistance.